Mechanics: State, evaluate and apply mechanical advantage
Unit 2: Pulleys and mechanical advantage
Leigh Kleynhans
Unit outcomes
By the end of this unit you will be able to:
- Define a pulley.
- Distinguish between fixed and movable pulleys.
- Identify and describe the mechanical advantage of a pulley system.
- Analyse and apply law (equation) of simple machines on pulleys (hoist) to determine the mechanical advantage.
What you should know
Before you start this unit, make sure you can:
- Identify, describe, and apply principles of simple machines and mechanical advantage. See level 2 subject outcome 2.4 to revise this.
Introduction[1]
In this unit, you will learn how pulleys can act as simple machines and provide a mechanical advantage. Thousands of years ago and still to this day, engineers have used pulleys to make everyday tasks and large construction projects easier. They use pulleys to raise heavy objects, taking advantage of gravity to help lift an object. Engineers incorporate pulleys into a wide range of modern-day applications, such as cranes for lifting very heavy objects in construction projects, elevators, flagpoles, motors, bicycles, and boats. To gain a mechanical advantage using multiple pulleys in conjunction with motors and electronics, engineers create devices that operate with very little power in relation to how much work they perform.
What is a pulley?
A is a simple machine that consists of a wheel with a groove, running along its circumference between the rims of the wheel. A rope or cable passes through the groove, which is attached to the load on one end, and the effort is applied to perform work at the other end. A is one that is attached to a non-moving point (an anchor) and the rope is attached to the object that needs to be raised or lowered. In a the rope is attached to a non-moving point and the pulley is attached to the object and which is able to slide along the rope.
Fixed pulleys
A fixed pulley is useful for raising an object to a level above your head. Using this type of pulley enables you to take advantage of gravity. A real-life example of this would be raising a flag on a flagpole.

The pulley in figure 1 redirects the force. This means that by looping a rope around the pulley and attaching the rope to an object, one pulls down on the rope to raise the object, instead of having to lift the object. Although this is a helpful and convenient use for pulleys, it has a major limitation: the force you must apply to lift the object is the same as if you were just lifting the object without the pulley. This means that a fixed pulley does not give any mechanical advantage.
By attaching a to the end of the rope that you pull, you can lessen the amount of force you must apply. Once a force is applied to either side, the system continues moving in that direction. This kind of pulley system is used in lifts. The lift has a cable attached to it that goes up, around a pulley, then comes down and attaches to a counterweight. The motor that moves the lift uses much less power since the counterweight provides a gravitational force.
Movable pulleys
In a movable pulley system, the rope is attached to a fixed (non-moving) point, the pulley is attached to the object that you want to move, and the other end of the rope is left free (see figure 2). By pulling on the rope, the pulley moves, and the object is raised.
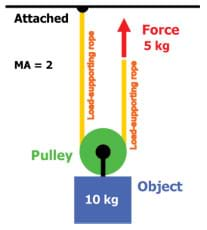
This type of system is good if you are trying to raise an object located below you to your level. In a variation, if both sides of a movable pulley system are fixed and the rope is taut between the fixed points, the system becomes like a wheel and axle because the object can ride along the rope if a force is applied to it (for example, a zip line).
Exercise 2.1
- Where have you seen pulleys before?
- Why do people use pulleys?
The full solutions are at the end of the unit.
The mechanical advantage of using multiple pulleys
How can pulleys make our lives easier? Pulleys are powerful simple machines. They can change the direction of a force, which can make it much easier for us to move something. If we want to lift an object that weighs [latex]\scriptsize 10\text{ kg}[/latex] to a height of [latex]\scriptsize 1\text{ m}[/latex], we can lift it straight up or we can use a pulley where we can pull down on one end to lift the object up. It is much easier to use the pulley because, as long as we weigh more than [latex]\scriptsize 10\text{ kg}[/latex], we can just hang onto the end of the rope and take advantage of gravity, so our weight provides all the necessary force to lift the object.
Pulleys can also provide us with a mechanical advantage when we use several together and more rope. This process lessens the amount of force required to lift something.
Activity 2.1: Demonstrate how using more rope can increase mechanical advantage
Time required: 10 minutes
What you need:
- 3 people
- a [latex]\scriptsize 6\text{ m}[/latex] piece of rope
- 2 brooms
What to do:
- Tie the rope to one of the brooms (broom 1) and wrap the rope around the other broom (broom 2). Have two people stand about a metre apart each holding a broom, and try to keep the brooms separated while the third person pulls on the free end of the rope; it should be a difficult task to pull the broom sticks together.
- Next, wrap the rope around each of the brooms again. Try again to pull the brooms together.
- Repeat the process again (see figure 3).
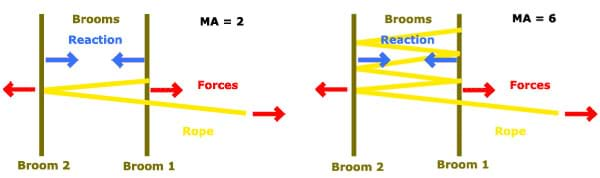
Figure 3: The arrangement of brooms and rope in step 2 and step 3
What did you find?
The more times you wrap the rope around the brooms, the easier it is for the third person to pull the brooms together. This is an example showing the power of mechanical advantage.
Combining multiple pulleys decreases the amount of force necessary to move an object by increasing the amount of rope used to raise the object. The mechanical advantage (MA) of a pulley system is equal to the number of ropes supporting the movable load. (Do not count ropes that are only used for redirecting the force.)
[latex]\scriptsize MA=\text{number of load supporting ropes}[/latex]
A single pulley therefore has a mechanical advantage of 1, but using multiple pulleys will proportionally increase the mechanical advantage.
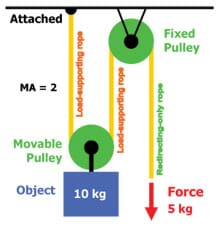
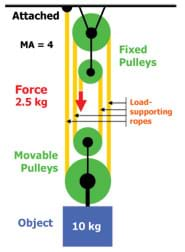
A system of multiple pulleys that creates a significant mechanical advantage is referred to as a . Engineers combine many pulleys into a pulley system that significantly reduces the amount of force required to lift an object. They often use pulley systems to move extremely heavy objects. A block and tackle system may take a lot of cable or rope, but a human using enough pulleys could lift several tons. Engineers use the block and tackle along with motors and electronics to create modern devices that operate with very low power requirements, such as cranes and elevators.
Exercise 2.2
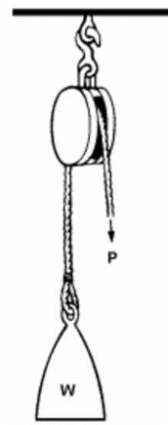
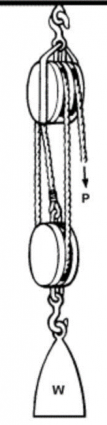
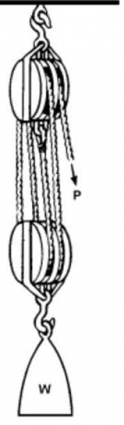
Determine the mechanical advantage of the following pulley systems:
- .
- .
- .
The full solutions are at the end of the unit.
Activity 2.2: Investigate the mechanical advantage of using multiple pulleys
Time required: 10 minutes
What you need:
- a plastic milk bottle filled with sand
- rope or string
What to do:
- Tie the rope to a strong bar (or rod) above your head (a playground swing works well).
- Run the rope through the handle of the milk bottle and then over the bar overhead.
- Raise the milk bottle by pulling the rope down. Note how difficult it is to do this.
- Next, run the rope through the milk bottle and over the bar again. Raise the milk bottle again.
- Continue to loop the rope through the milk bottle and over the bar noting how much easier it becomes to raise the milk bottle . (See figure 6)
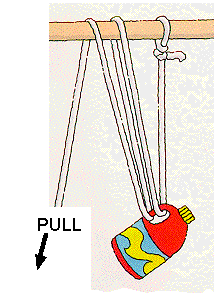
Figure 6: A milk bottle being raised by multiple pulleys
What did you find?
- Each time the rope is looped over the rod, the force required to lift the bottle becomes less.
- This is an example of a block and tackle system of multiple pulleys.
- Even though proper pulleys are not used, the mechanical advantage of increasing the length of the rope and having the rope support the object being raised multiple times demonstrates the mechanical advantage of the system.
- Using real pulleys would decrease the friction of the system and therefore make the process even easier.
Note
Watch this video of an experiment to demonstrate the mechanical advantage of multiple pulleys.
Summary
In this unit you have learnt the following:
- A pulley is a simple machine consisting of a grooved wheel in which a pulled rope or chain runs.
- A pulley changes the direction of a force to lift a load.
- Pulleys are divided into two types: a fixed pulley is attached to a non-moving point and the rope is attached to the object, while a movable pulley is attached to the object.
- Using a single pulley does not give any mechanical advantage, however using several pulleys together can create a mechanical advantage.
- The mechanical advantage of a system of pulleys reduces the effort by increasing the length of the rope used in the system.
- The equation to calculate the mechanical advantage of a pulley system is:
[latex]\scriptsize MA=\text{number of load supporting ropes}[/latex] - Multiple pulley systems, called a block and tackle, are used to reduce the effort required to hoist very heavy objects.
Unit 2: Assessment
Suggested time to complete: 20 minutes
- Define a pulley
- Name the two kinds of pulleys and explain the difference between them.
- Explain how to find the mechanical advantage of a pulley system.
- What is the trade-off of mechanical advantage in a pulley system?
- What are some examples of modern items that engineers have designed with pulleys?
- A rock of [latex]\scriptsize 100\text{ kg}[/latex] needs to be raised.
- How much force would be required if a single pulley was used?
- How much force would be required if a pulley system with five supporting ropes was used?
- The engine of a vehicle needs to be raised by a block and tackle with four supporting chains. The force used is [latex]\scriptsize 5\text{00 N}[/latex]. Calculate the mass of the engine.
- A single pulley does not provide a mechanical advantage ([latex]\scriptsize MA=1[/latex]).
- Explain this statement.
- What would be the benefit of using a single pulley to raise a heavy object?
The full solutions are at the end of the unit.
Unit 2: Solutions
Exercise 2.1
- Possible answers: Crane, flagpole, blinds or shades, gym equipment, clothesline, water well, zip line, bike chain, elevator, sailboats.
- To change the direction of force or make it easier to lift something.
Exercise 2.2
- [latex]\scriptsize MA=\text{number of load supporting ropes = 1}[/latex]
- [latex]\scriptsize MA=\text{number of load supporting ropes = 3}[/latex]
- [latex]\scriptsize MA=\text{number of load supporting ropes = 4}[/latex]
Unit 2: Assessment
- A pulley is a simple machine consisting of a grooved wheel in which a pulled rope or chain runs.
- A fixed pulley is attached to a fixed point and the rope is attached to the object. A movable pulley is attached to the object with one end of the rope attached to a fixed point and the other end of the rope is free.
- The mechanical advantage of a pulley is system is equal to the number of ropes supporting the object being lifted or hauled.
- The greater the mechanical advantage the greater the length of rope required.
- Possible answers: Cranes, elevators, block and tackle on boats, flagpoles, zip lines, motors, bicycle rings/chains, rock climbing devices, window blinds and sail boats.
- .
- .
[latex]\scriptsize \begin{align*}\text{Force required to lift 100 kg}&=m\text{ x }g\text{ = 100 x 9}\text{.8 = 980N}\\MA &=\text{no}\text{. of supporting ropes = 1}\\&\text{980 N must be force applied}\end{align*}[/latex] - .
[latex]\scriptsize \begin{align*}\text{Force required to lift 100 kg}&=m\text{ x }g\text{ = 100 x 9}\text{.8 = 980N}\\MA&=\text{no}\text{. of supporting ropes = 5}\\\text{980 }\div \text{ 5 }&= \text{196 N must be force applied}\end{align*}[/latex]
- .
- .
[latex]\scriptsize \begin{align*}MA&=\text{ no}\text{. of supporting ropes = 4}\\\text{lifting force }&= \text{4 x applied force = 4 x 500 = 2 000 N}\\m&=\displaystyle \frac{F}{g}=\displaystyle \frac{{2\text{ }000}}{{9.8}}=204.08\text{ kg}\end{align*}[/latex] - .
- The force applied (effort) is the same as the force required to raise the object (load).
- A pulley can redirect the force so instead of pulling upwards, you can pull downwards which is easier to do because it is with gravity rather than against gravity.
Media Attributions
- img01_Figure1 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img02_Figure2 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img03_Figure3 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img04_Figure4 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img05_Figure5 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img06_Ex2.2Q1 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img07_Ex2.2Q2 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img08_Ex2.2Q3 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- img09_Figure6 © Carlson, Sullivan, Fritts & Zarske is licensed under a CC BY (Attribution) license
- Parts of the text in this unit were sourced from Teach Engineering released under a CC-BY licence. ↵
a simple machine consisting of a grooved wheel in which a pulled rope or chain runs
a pulley system in which the pulley is attached to a fixed point and the rope is attached to the object
a pulley system in which the pulley is attached to the object; one end of the rope is attached to a fixed point and the other end of the rope is free
a weight that, by applying an opposite force, provides balance and stability in a mechanical system
an apparatus of pulleys and ropes used for hauling and hoisting heavy objects
