Matter and Materials: Identify and critically evaluate the impact of atomic nuclei on the quality of human, environmental and socio-economic development
Unit 1: Radioactivity
Emma Harrage
Unit outcomes
By the end of this unit you will be able to:
- Describe and define the nuclear structure of an atom and its stability.
- Explain radioactivity and how it is measured.
- Understand nuclear fusion and fission and nuclear power.
What you should know
Before you start this unit, make sure you:
- Understand isotopes. Refer to level 2 subject outcome 5.2 unit 2 if you need help with this.
Introduction[1]
In this unit you will learn about radioactivity. Radioactivity occurs when a nucleus in an atom is unstable because of the number of protons and neutrons. We are exposed to radiation every day from the sun – the sun is a giant ball of gases which produces light and heat because of nuclear fusion. Radioactive elements such as uranium are used to generate electricity. Over exposure to radiation can cause you to develop radiation sickness and cancers.
Atoms and stability
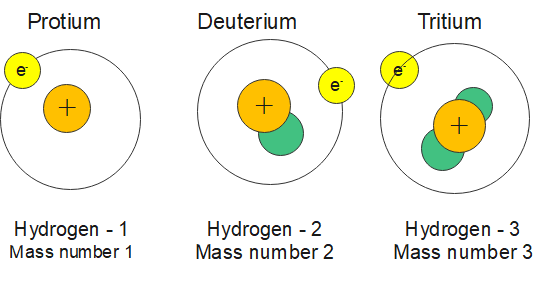
As we learnt in level 2 subject outcome 5.2 unit 2, atoms are composed of subatomic particles – protons, neutrons, and electrons. Protons and neutrons are in the nucleus and provide most of the mass of the atom, while electrons circle the nucleus in shells and subshells and account for an atom’s size. Remember, the notation for representing an isotope of a particular atom is as follows:
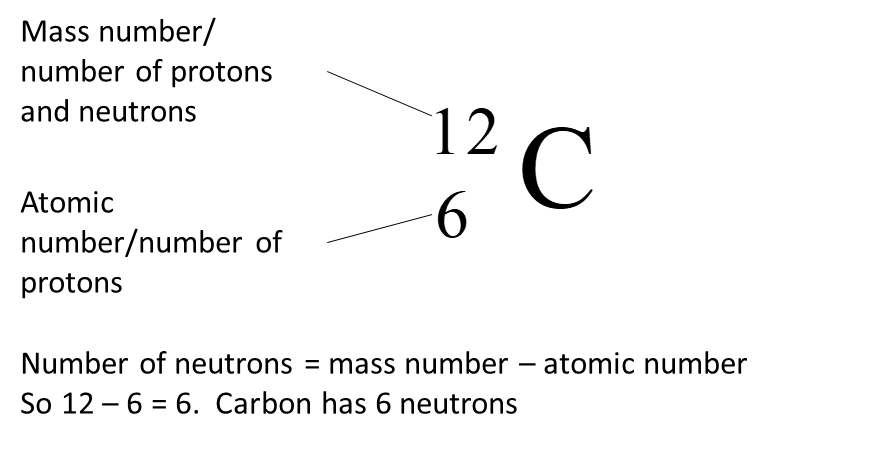
An atom is stable because of a balanced nucleus that does not contain excess energy. If the forces between the protons and the neutrons in the nucleus are unbalanced, then the atom is unstable. Stable atoms retain their form indefinitely, while unstable atoms undergo . Most naturally occurring atoms are stable and do not decay.
Unstable atoms are and decay after a certain amount of time. Different unstable atoms decay through different processes, such as the discharge of a proton or a neutron or the conversion of a proton to a neutron or a neutron to a proton. This result causes the atom to remain unstable until it decays to form a new stable atom.
Most elements have isotopes; in many cases one or more of these isotopes is radioactive, whilst others are not radioactive. For this reason, it is usual to refer to individual isotopes when describing radioactivity. e.g. cobalt-[latex]\scriptsize \displaystyle 60[/latex] is radioactive, but cobalt-[latex]\scriptsize \displaystyle 59[/latex] is not.
Major types of radioactivity
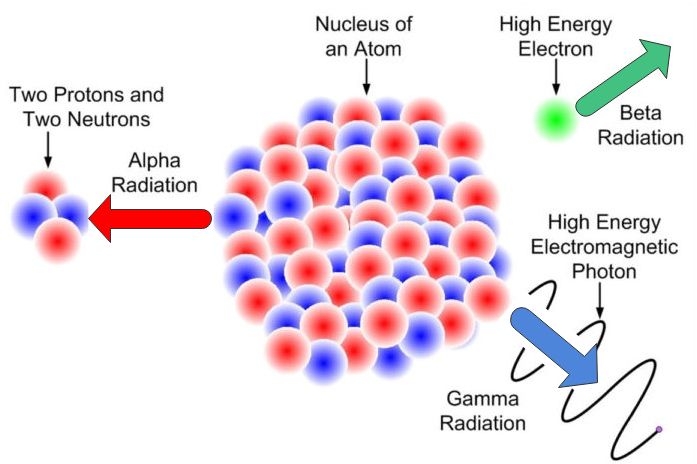
Atomic theory in the 19th century presumed that nuclei had fixed compositions. But in 1896, the French scientist Henri Becquerel found that a uranium compound placed near a photographic plate made an image on the plate, even if the compound was wrapped in black cloth. He reasoned that the uranium compound was emitting some kind of radiation that passed through the cloth to expose the photographic plate. Further investigations showed that the radiation was a combination of particles and electromagnetic rays, from the atomic nucleus. These rays were collectively called . There are three main forms of radioactive emissions.
Alpha ([latex]\scriptsize \alpha[/latex]) particles
The first is called an alpha particle, which is symbolised by the Greek letter [latex]\scriptsize \alpha[/latex]. An alpha particle is composed of two protons and two neutrons, and so it is the same as a helium nucleus: [latex]\scriptsize _{2}^{4}\text{He}[/latex].
When a radioactive atom emits an alpha particle, the original atom’s atomic number decreases by two (because of the loss of two protons), and its mass number decreases by four (because of the loss of 2 protons and 2 neutrons). We can represent the emission of an alpha particle with a chemical equation. For example, the alpha particle emission of uranium, [latex]\scriptsize _{{}}^{{235}}\text{U}[/latex], is as follows:
[latex]\scriptsize _{{92}}^{{235}}\text{U}\to _{2}^{4}\text{He}+\text{ }_{{90}}^{{231}}\text{Th}[/latex]
How do we know that a product of the reaction is [latex]\scriptsize _{{90}}^{{231}}\text{Th}[/latex]?
We use the law of conservation of matter, which says that matter cannot be created or destroyed. This means there must be the same number of protons and neutrons on both sides of the chemical equation.
If the uranium nucleus loses [latex]\scriptsize \displaystyle 2[/latex] protons, there are [latex]\scriptsize \displaystyle 90[/latex] protons remaining, identifying the element as thorium. If we lose [latex]\scriptsize \displaystyle 4[/latex] nuclear particles of the original [latex]\scriptsize \displaystyle 235[/latex], there are [latex]\scriptsize \displaystyle 231[/latex] remaining. Thus, we use subtraction to identify the isotope of the thorium atom, [latex]\scriptsize _{{90}}^{{231}}\text{Th}[/latex] .
When one element changes into another in this manner, it undergoes .
Radon has an atomic number of [latex]\scriptsize \displaystyle 86[/latex], so the isotope is represented as [latex]\scriptsize _{{86}}^{{222}}\text{Rn}[/latex]. We represent the alpha particle as [latex]\scriptsize _{2}^{4}\text{He}[/latex] and use subtraction:
[latex]\scriptsize \displaystyle \begin{align*}222-4&=218\text{ }\\86-2&=84\end{align*}[/latex]
We can now identify the decayed isotope as an isotope of polonium, [latex]\scriptsize _{{84}}^{{218}}\text{Po}[/latex]:
[latex]\scriptsize _{{86}}^{{222}}\text{Rn}\to _{2}^{4}\text{He}+_{{84}}^{{218}}\text{Po}[/latex]
Beta ([latex]\scriptsize \text{ }\!\!\beta\!\!\text{ }[/latex]) particles
The second major type of radioactive emission is called a beta particle, symbolised by the Greek letter [latex]\scriptsize \text{ }\!\!\beta\!\!\text{ }[/latex]. A beta particle is an electron ejected from the nucleus (not from the shells of electrons about the nucleus). We can also represent a beta particle as [latex]\scriptsize \displaystyle ~_{{-1}}^{0}e\ \ or \ \ \!\!\beta\!\!\text{ }-.[/latex] The net effect of beta particle emission on a nucleus is that a neutron is converted to a proton. The overall mass number stays the same, but because the number of protons increases by one, the atomic number goes up by one. Carbon-14 decays by emitting a beta particle:
[latex]\scriptsize \displaystyle _{6}^{{14}}\text{C}\to _{7}^{{14}}\text{N}+_{{-1}}^{0}e[/latex]
Again, the sum of the atomic numbers is the same on both sides of the equation, as is the sum of the mass numbers. Note that the electron is assigned an ‘atomic number’ of [latex]\scriptsize \displaystyle -1[/latex], equal to its charge.
The emission of an α-particle or a β-particle from a nucleus is an example of a . A nuclear reaction results in the change in the composition of a nucleus and therefore results in the formation of new atoms with different atomic numbers. Chemical reactions, by contrast, do not change the composition of a nucleus – they only involve the rearrangement of the electrons in orbitals and therefore do not result in the formation of new atoms.
For this reason, nuclear equations always include atomic numbers and mass numbers of every particle, as they change during the reaction.
Gamma ([latex]\scriptsize \gamma[/latex]) rays
The third major type of radioactive emission is not a particle but rather a very energetic form of electromagnetic radiation called gamma rays, symbolised by the Greek letter[latex]\scriptsize \gamma[/latex]. Gamma rays themselves do not carry an overall electrical charge, but they may knock electrons out of atoms in a sample of matter and make it electrically charged.
For example, in the radioactive decay of radon, [latex]\scriptsize _{{}}^{{222}}\text{Ra}[/latex], both alpha and gamma radiation are emitted, with the latter having an energy of [latex]\scriptsize \displaystyle 8.2\text{ }\times \text{ }{{10}^{{-14}}}\text{ J}[/latex] per nucleus decayed. This may not seem like much energy, but if [latex]\scriptsize \displaystyle 1\text{ mol}[/latex] of radon atoms were to decay, the gamma ray energy would be [latex]\scriptsize \displaystyle 49[/latex] million kJ (which is the same amount of energy in [latex]\scriptsize \displaystyle 82\text{ }000[/latex] bananas!).
[latex]\scriptsize \displaystyle _{{86}}^{{222}}\text{Rn}\to _{{84}}^{{218}}\text{Po}+_{2}^{4}\text{He}+\gamma[/latex]
The emission of an [latex]\scriptsize \displaystyle \alpha[/latex]– or [latex]\scriptsize \displaystyle \text{ }\!\!\beta\!\!\text{ }[/latex]-particle often results in a much more stable nucleus with a much lower potential energy; the potential energy is converted into a high-energy photon of electromagnetic gamma radiation. Gamma rays are only emitted at the same time as [latex]\scriptsize \displaystyle \text{ }\!\!\alpha\!\!\text{ }[/latex]– or [latex]\scriptsize \displaystyle \text{ }\!\!\beta\!\!\text{ }[/latex]-particles.
Below is a summary of the three main forms of radioactive emissions
| Type | Symbol | Radiation emitted | Description |
| Alpha | [latex]\scriptsize _{\text{2}}^{\text{4}}\text{He or }_{2}^{4}\text{ }\!\!\alpha\!\!\text{ }[/latex] | [latex]\scriptsize _{2}^{4}\text{ }\!\!\alpha\!\!\text{ }[/latex] | High energy helium nuclei consisting of two protons and two neutrons. It has a large mass, compared to other ionising radiations, and a strong positive charge. |
| Beta | [latex]\scriptsize _{{\text{-1}}}^{\text{0}}\text{e or }_{{-1}}^{0}\text{ }\!\!\beta\!\!\text{ }[/latex] | [latex]\scriptsize \text{ }_{{-1}}^{0}\text{ }\!\!\beta\!\!\text{ }[/latex] | High energy electrons. It has a very small mass and a negative charge. |
| Gamma | γ | [latex]\scriptsize _{0}^{0}\text{ }\!\!\gamma\!\!\text{ }[/latex] | Very high energy electromagnetic radiation. Gamma rays are caused by changes within the nucleus. They are part of the electromagnetic spectrum and so travel at the speed of light. They have no mass and no charge. |
Exercise 1.1
- .
- Describe an alpha particle; explain what happens to a nucleus after it emits an alpha particle.
- Describe a beta particle; explain what happens to a nucleus after it emits a beta particle.
- Explain why gamma rays are sometimes emitted at the same time as an alpha or beta particle
- Write the nuclear equation that represents the radioactive decay of polonium-209 by alpha particle emission and identify the decayed isotope.
The full solutions are at the end of the unit.
Properties of radiation
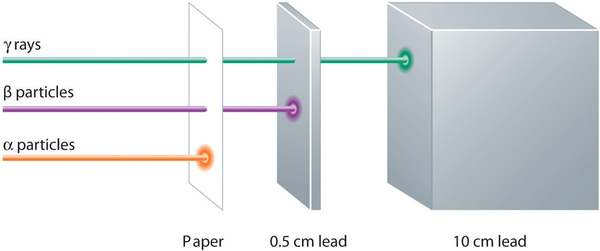
Alpha particles collide easily with other particles, pulling electrons away from them until the [latex]\scriptsize \displaystyle \alpha[/latex]-particle has gained two electrons and become a stable helium atom. As a result [latex]\scriptsize \displaystyle \alpha[/latex]-particles are very strongly – any particles they hit are likely to lose electrons and become ionised. Because of this, [latex]\scriptsize \displaystyle \alpha[/latex]-particles are very quickly destroyed, and they generally travel no further than [latex]\scriptsize \displaystyle 4~\text{cm}[/latex] in air and are easily stopped by a thin piece of paper.
Beta particles are much smaller and so collide less easily with other particles. When they do collide with other particles, they transfer energy to them, and this can result in other electrons being lost from that atom. As a result [latex]\scriptsize \displaystyle \text{ }\!\!\beta\!\!\text{ }[/latex]-particles are also ionising, although much less so than [latex]\scriptsize \displaystyle \alpha[/latex]-particles; eventually the [latex]\scriptsize \displaystyle \text{ }\!\!\beta\!\!\text{ }[/latex]-particle will slow down and be absorbed into the electron shells of another atom. [latex]\scriptsize \displaystyle \text{ }\!\!\beta\!\!\text{ }[/latex]-particles have more penetrating power than [latex]\scriptsize \displaystyle \alpha[/latex]-particles; they can travel a long distance through air and can pass through paper but can be stopped by a thin sheet of metal.
Gamma rays have no charge and no mass, they therefore do not cause in other particles and often pass through them completely without being absorbed. As a result, [latex]\scriptsize \gamma[/latex]-rays have a very low ionising power but a very high penetrating power. They cannot be completely stopped and a few centimetres of lead or several metres of concrete is needed to significantly reduce their penetration.
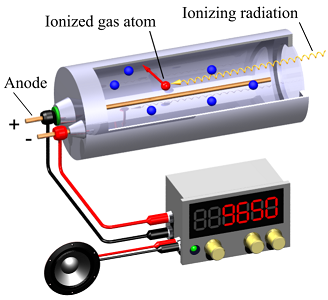
Detecting radiation
The ionising properties of radiation mean that it can be detected by a piece of equipment known as a Geiger Muller tube. This tube contains gaseous particles which become ionised by the radiation, creating a current which can be detected; each radioactive particle or ray produces one pulse. The total concentration of radiation in a particular location can be measured from the number of pulses per second; a Geiger-Muller tube does not distinguish between alpha, beta, and gamma radiation.
All three types of radiation are high in energy and if living cells are exposed to significant quantities of radiation, they can be seriously damaged. Sometimes the cells are killed and sometimes the cells will mutate and become cancerous; either way, high levels of exposure to radiation can be fatal.
The relative dangers of alpha, beta and gamma radiation are directly linked to their ionising power, their penetrating power and how the exposure takes place:
- Alpha and beta radiation is highly ionising but cannot penetrate skin; external alpha and beta radiation is therefore not considered dangerous unless it is present in large quantities and very close, in which case it will kill skin cells (burn the skin). If radioactive atoms are ingested, injected, or inhaled, however, they can be very dangerous as they release ionising radiation inside the body; this can kill healthy cells or turn them into cancerous cells.
- Gamma radiation can pass through the body due to its low ionising power; small quantities of gamma radiation are not considered dangerous. In fact, small quantities of gamma radiation can come from space and are emitted by rocks and soil. However, large quantities of gamma radiation can be dangerous wherever it is, and you can be harmed or killed by gamma radiation without inhaling, injecting, or ingesting radioactive material.
Radioactive decay
The rate of chemical reactions depends on various factors including temperature, pressure or concentration, surface area and the presence of a catalyst. The rate at which a nucleus emits [latex]\scriptsize \displaystyle \alpha[/latex]-particles and [latex]\scriptsize \displaystyle ~\text{ }\!\!\beta\!\!\text{ }[/latex] -particles does not depend on temperature, surface area or any catalyst, it depends on how many radioactive atoms are present in the sample. Nuclear decay reactions occur spontaneously under all conditions and produce more stable daughter nuclei, whereas nuclear transmutation reactions are induced and form a product nucleus that is more massive than the starting material.
The rate of radioactive decay of a particular isotope is directly proportional to the number of atoms of that isotope in the sample.
As a result of this, the time taken for half of a sample radioactive isotope to decay is fixed for every radioactive isotope. The time taken for half of the atoms to decay is called the of that isotope and the half-life of radioactive isotopes can vary from fractions of a second to millions of years.
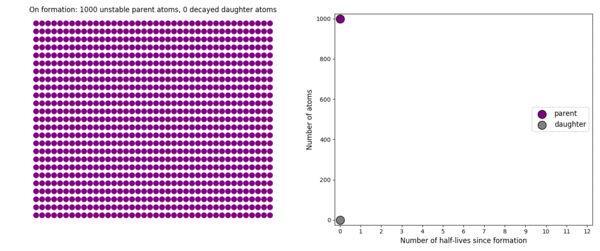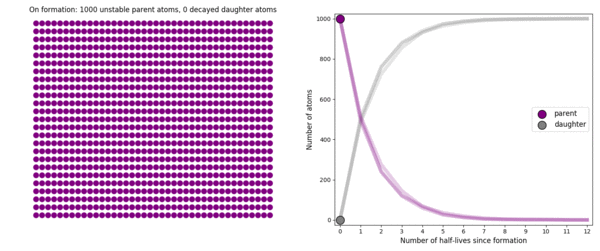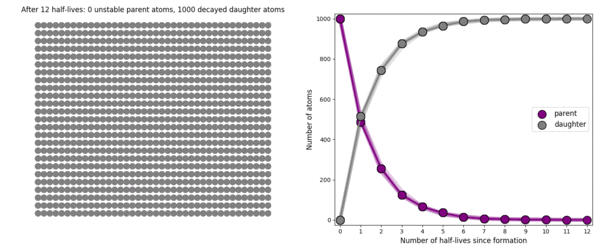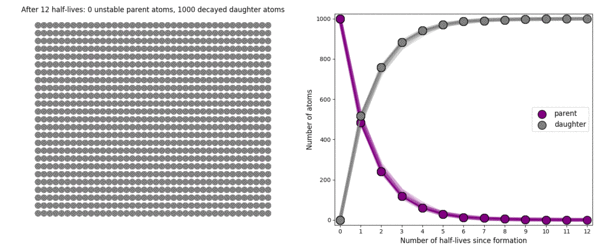
| Isotope | Half-life |
| [latex]\scriptsize _{{}}^{{90}}\text{Kr}[/latex] | [latex]\scriptsize 33\text{ s}[/latex] |
| [latex]\scriptsize _{{}}^{{108}}\text{Ag}[/latex] | [latex]\scriptsize 2.4\min[/latex] |
| [latex]\scriptsize _{{}}^{{131}}\text{I}[/latex] | [latex]\scriptsize 8\text{ days}[/latex] |
| [latex]\scriptsize _{{}}^{{14}}\text{C}[/latex] | [latex]\scriptsize 5730\text{ years}[/latex] |
| [latex]\scriptsize _{{}}^{{238}}\text{U}[/latex] | [latex]\scriptsize 4.46\text{ billion years}[/latex] |
After one half-life, [latex]\scriptsize \displaystyle 50\%[/latex] of a sample will have decayed and [latex]\scriptsize \displaystyle 50\%[/latex] will be remaining; after two half-lives, [latex]\scriptsize \displaystyle 75\%[/latex] of a sample will have decayed and [latex]\scriptsize \displaystyle 25\%[/latex] will be remaining; after three half-lives, [latex]\scriptsize \displaystyle 87.5\%[/latex] of a sample will have decayed and [latex]\scriptsize \displaystyle 12.5\%[/latex] will be remaining.
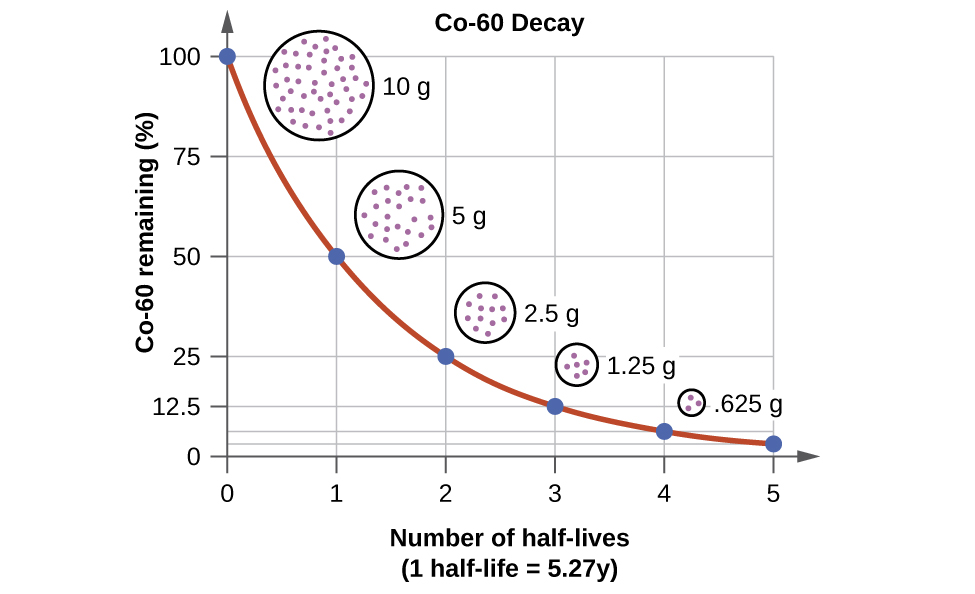
Example 1.1
How long will it take for [latex]\scriptsize \displaystyle 18.0[/latex] grams of [latex]\scriptsize _{{}}^{{226}}\text{Ra}[/latex] to decay to leave a total of [latex]\scriptsize \displaystyle 2.25[/latex] grams? [latex]\scriptsize _{{}}^{{226}}\text{Ra}[/latex] has a half-life of [latex]\scriptsize \displaystyle 1600[/latex] years.
Solution
[latex]\scriptsize \displaystyle 18.0\text{ g}\Rightarrow 9.0\text{ g}\Rightarrow 4.5\text{ g}\Rightarrow 2.25\text{ g}[/latex], this is three half-lives.
(time) =[latex]\scriptsize \displaystyle 3\times 1600[/latex] years
This decay process takes [latex]\scriptsize \displaystyle 4800[/latex] years to occur.
Example 1.2
[latex]\scriptsize _{{}}^{{60}}\text{Co}[/latex] has a half-life of [latex]\scriptsize \displaystyle 5.3[/latex] years; starting with [latex]\scriptsize \displaystyle 1000[/latex] atoms, how many atoms will have decayed after [latex]\scriptsize \displaystyle 10.6[/latex] years and how many will remain?
Solution
[latex]\scriptsize \displaystyle 10.6[/latex] years = [latex]\scriptsize \displaystyle \displaystyle \frac{{10.6}}{{5.3}}=\text{ }2[/latex] half-lives, so [latex]\scriptsize \displaystyle 75\%[/latex] or [latex]\scriptsize \displaystyle 750[/latex] atoms will have decayed and [latex]\scriptsize \displaystyle 25\%[/latex] or [latex]\scriptsize 250[/latex] atoms will be remaining .
Example 1.3
A sample of Ac –[latex]\scriptsize \displaystyle 225[/latex] originally contained [latex]\scriptsize \displaystyle 8.0\text{ ug}[/latex]. After [latex]\scriptsize \displaystyle 720[/latex] hours, how much of the original Ac –[latex]\scriptsize \displaystyle 225[/latex] remains? The half-life of this isotope is [latex]\scriptsize \displaystyle 10[/latex] days.
Solution
To determine the number of half-lives (n), both time units must be the same:
[latex]\scriptsize \displaystyle \begin{align*}{*{20}{l}} {720\text{ hours}\times \displaystyle \frac{{1\text{ day}}}{{24\text{ hours}}}=30\text{ days}} \\ {\text{n}=3=\displaystyle \frac{{30\text{ days}}}{{10\text{ days}}}} \end{align*}[/latex]
How much mass remains = [latex]\scriptsize \displaystyle \displaystyle \frac{1}{{{{2}^{3}}}}\left( {8.0\text{ ug}} \right)[/latex]
After [latex]\scriptsize \displaystyle 720[/latex] hours, [latex]\scriptsize \displaystyle 1.0\text{ ug}[/latex] of the material remains as Ac –[latex]\scriptsize \displaystyle 225[/latex].
The more stable a nucleus, the longer its half-life; the less stable a nucleus, the shorter its half-life. Nuclei which are completely stable (i.e. non-radioactive) do not have a half-life and have an infinite lifetime.
Radioactive isotopes with a long half-life are considered more of an environmental hazard than those with a short half-life, as they remain active in the environment for a much longer time.
Exercise 1.2
- Define the term ‘half-life’.
- [latex]\scriptsize _{{}}^{{108}}\text{Ag}[/latex] has a half-life of [latex]\scriptsize \displaystyle 2.4[/latex] minutes; calculate the percentage of a sample which will have decayed after [latex]\scriptsize \displaystyle 7.2[/latex] minutes.
The full solutions can be found at the end of the unit.
Nuclear reactions and nuclear energy
The emission of an α-particle or a β-particle from a nucleus (radioactive decay) is just one example of a nuclear reaction; there are two other important types of nuclear reactions, nuclear fission and nuclear fusion.
Nuclear fission
is the break-up of a large nucleus to form two or more smaller nuclei; it does not occur spontaneously and only happens when a nucleus is bombarded with a neutron.
E.g.: [latex]\scriptsize \displaystyle _{{92}}^{{235}}\text{U}+_{0}^{1}\text{n }\to _{{56}}^{{141}}\text{Ba + }_{{36}}^{{92}}\text{Kr +3}_{0}^{1}\text{n}[/latex]
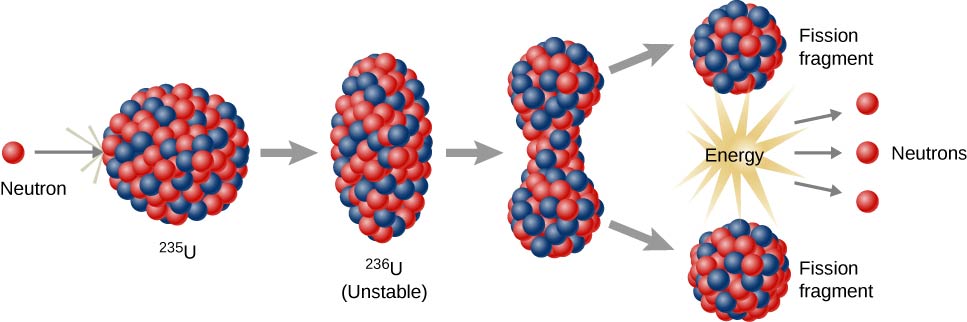
The two smaller nuclei are usually more stable than the original large nucleus, so a lot of heat energy and gamma rays are released during nuclear fission; in addition, the neutrons produced can collide with more [latex]\scriptsize \displaystyle _{{}}^{{235}}\text{U}[/latex] atoms and cause further fission, starting a nuclear chain reaction.
During nuclear fission, the nucleus usually divides asymmetrically rather than into two equal parts, as shown in figure 7. In addition, every fission event of a given nuclide does not give the same products; more than [latex]\scriptsize \displaystyle 50[/latex] different fission modes have been identified for uranium-[latex]\scriptsize \displaystyle 235[/latex], for example. Consequently, nuclear fission of a fissile nuclide can never be described by a single equation.
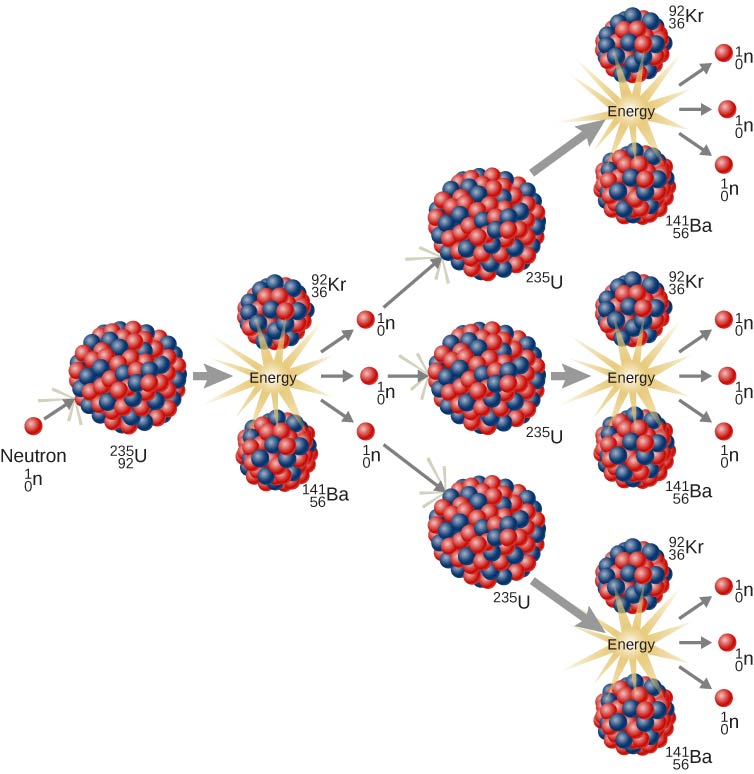
As shown in figure 8, the collision of a relatively slow-moving neutron with a fissile nucleus can split it into two smaller nuclei with the same or different masses. Neutrons are also released in the process, along with a great deal of energy.
Most nuclear fission reactions are man-made, and the products are often radioactive isotopes; radiation emitted from isotopes produced by man-made nuclear reactions is called ‘artificial radiation’.
Nuclear fission is carried out in nuclear reactors to produce nuclear energy. Most nuclear reactors use [latex]\scriptsize _{{}}^{{235}}\text{U}[/latex] which is present in small quantities in naturally occurring uranium. The uranium needs to be ‘enriched’, which means increasing the amount of [latex]\scriptsize _{{}}^{{235}}\text{U}[/latex], before it is used in nuclear reactors. This is done by bombarding the nucleus with neutrons and break up (i.e. the nuclei undergo nuclear fission). This produces a large amount of heat as well as extra neutrons. The excess heat needs to be cooled, and this is usually done with water.

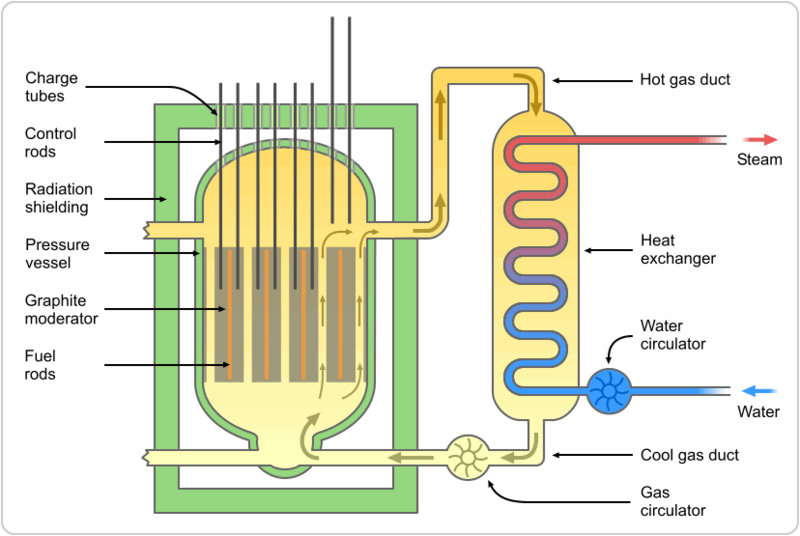
In a nuclear power station nuclear fuel undergoes a controlled chain reaction in the reactor to produce heat – nuclear energy to heat energy. The chain reaction is controlled by boron control rods. Boron is a non-fissile material. This means it will not undergo a fission reaction when bombarded with neutrons. When the Boron absorbs the neutrons then the chain reaction will slow down due to a lack of neutron-producing reactions.
In times of high energy demand, control rods are raised allowing more reactions to take place. In times of low demand, control rods are lowered to absorb neutrons and hence decrease the number of reactions; less reactions = less heat energy produced.
Heat from the nuclear reaction is used to change water into steam in the boiler. (The water in the boiler NEVER actually comes into contact with the same coolant that is used in the reactor but is transferred from the reactor to the external water by using a heat exchanger.)
The steam drives the turbine (heat energy to kinetic energy – the same as a conventional power station).
This drives the generator to produce electricity – kinetic energy to electrical energy.
- The fuel rods: source of enriched uranium fuel that undergoes fission reactions.
- The moderator: the fuel rods are encased in the moderator (graphite is sometimes used) and help the fission reaction to proceed efficiently.
- The control rods: these are normally made of boron and help control the fission reaction.
- The coolant: this is a liquid or gas that transfers energy from the reactor to the heat exchanger; it’s also used to cool the reactor.
- The containment vessel: this is a thick concrete shield that absorbs neutrons and ionising radiation. In practice this can be [latex]\scriptsize \displaystyle 5.5\text{ m}[/latex] thick.
There are a number of advantages of nuclear fuels:
- Unlike fossil fuels, nuclear fuels do not produce carbon dioxide or sulphur dioxide.
- In terms of energy produced, [latex]\scriptsize \displaystyle 1\text{ kg}[/latex] of nuclear fuel produces the same energy as [latex]\scriptsize \displaystyle 2,900,000\text{ kg}[/latex] of coal.
- Since comparatively small volumes of fuel are used, it can be easily transported by road or rail.
- Waste products are small in volume.
There are also disadvantages of nuclear fuels:
- Like fossil fuels, nuclear fuels are non-renewable energy resources.
- If there is an accident, large amounts of radioactive material could be released into the environment. Modern reactor designs are extremely safe, and this very rarely happens.
- Nuclear waste remains radioactive and is hazardous to health for thousands of years. It must be stored safely. Storing nuclear waste is another hotly debated issue. Nuclear waste from Koeberg is stored in the Kalahari Desert.
- An atom bomb is a device which releases a large amount of energy very quickly as a result of a nuclear fission reaction, causing an explosion. Usually plutonium-[latex]\scriptsize \displaystyle 239[/latex] or uranium-[latex]\scriptsize \displaystyle 235[/latex] is used and the reaction is started by firing neutrons at the sample. Because nuclear fission produces radioactive isotopes as products, atom bombs can leave behind radioactive material long after they have exploded.
Nuclear fusion
is the joining together of two smaller nuclei to form a single, larger nucleus.
E.g. [latex]\scriptsize _{1}^{2}\text{H + }_{1}^{3}\text{H}\to _{2}^{4}\text{He + }_{0}^{1}\text{n}[/latex]
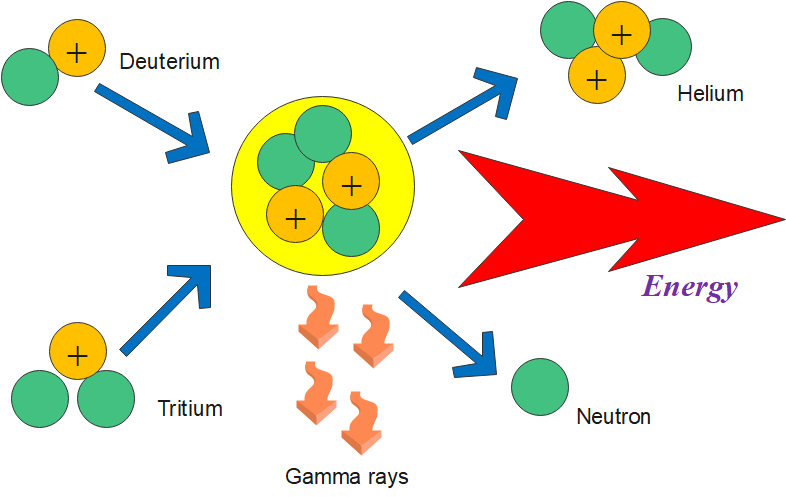
The larger nucleus is usually more stable than the two smaller nuclei, and so a lot of heat energy and gamma rays are released during nuclear fusion. Nuclear fusion takes place naturally in the sun – especially the fusion of hydrogen into helium. Nuclear fusion reactions are the source of the sun’s energy, and it is not currently possible to produce energy commercially using nuclear fusion reactions because very high temperatures are needed.
Nuclear fusion does not generally produce radioactive products; as a result it is considered much safer than nuclear fission.
A hydrogen bomb is a device which releases a large amount of energy very quickly because of the nuclear fusion of hydrogen atoms. Due to the large amount of energy required to start nuclear fusion, a nuclear fission reaction is used to create the heat necessary for the nuclear fusion reaction to start.
Note
To consolidate your understanding of fusion and fission you can watch this video by the US Department of Energy.
Exercise 1.3
- Explain the meaning of the term ‘nuclear fission’.
- Explain the meaning of the term ‘nuclear fusion’.
- Explain how electricity can be made from nuclear fission and explain why it is not currently possible to make electricity from nuclear fusion.
The full solutions are at the end of the unit.
The use of radioactivity
Although the radiation emitted by radioactive atoms can be dangerous, it also has useful applications, such as for carbon dating and as tracers.
Carbon-dating is done using carbon-[latex]\scriptsize \displaystyle 14[/latex], which is radioactive and has a half-life of [latex]\scriptsize \displaystyle 5730[/latex] years. Carbon atoms in living organisms are constantly being replaced due to the carbon cycle and so the proportion of carbon existing as carbon- [latex]\scriptsize \displaystyle 14[/latex] in living organisms is constant. When cells die, the carbon atoms in those cells are no longer replaced and so the amount of carbon-[latex]\scriptsize \displaystyle 14[/latex] in those cells gradually decreases over time. As the carbon-[latex]\scriptsize \displaystyle 14[/latex] decays [latex]\scriptsize \displaystyle 5730[/latex] years after death, it is possible to estimate how many years ago a sample of tissue died, and this can be used to estimate the age of fossils, skeletons, and some fabrics.
Radioactive material is used in tracers. Because gamma radiation can travel through walls and earth, it is possible to track the location of gamma emitting radioactive material by detecting the gamma rays. Tracers are used in several ways:
- Tracers are used in industry to locate blockages in underground or underwater pipes, a sample of radioactive material is inserted into the pipe and its progress is followed by tracking the radiation it emits. Radiation will accumulate at a blockage and disperse at a leak so engineers can find leaks and blockages without opening up the entire pipe.
- Tracers are used in agriculture to monitor how, and how fast, plants can absorb certain nutrients from the soil. Plants absorb water and other elements from the soil; if radioactive isotopes of these elements are placed in the soil, their rate of absorption can be measured by how much radioactivity is detected in the plant.
- Tracers are used in medicine to monitor the digestive system, respiratory system, or circulatory system; radioactive material can be inhaled, ingested, or injected and its progress through the body can be monitored.
- Cancer cells also absorb certain atoms (for example thyroid cancer absorbs iodine) so radioactive iodine will concentrate in the cancerous area, allowing the cancer to be located and treated. Exposure to large quantities of radiation is known to kill cells or cause them to mutate and become cancerous, but radiation can also be used to kill cancerous cells by using a targeted dose of radiation without harming healthy cells.
Summary
In this unit you have learnt the following:
- The major types of radioactivity include alpha particles, beta particles, and gamma rays.
- Alpha decay is a type of radioactive decay in which a particle with two neutrons and two protons is ejected from the nucleus of a radioactive atom.
- Beta decay is a type of radioactive decay in which a beta particle (fast energetic electron or positron) is emitted from an atomic nucleus.
- Gamma decay is a type of radioactive decay that a nucleus can undergo. What separates this type of decay process from alpha or beta decay is that no particles are ejected from the nucleus when it undergoes this type of decay. Instead, a high energy form of electromagnetic radiation – a gamma ray photon – Is released.
- The half-life of an isotope is used to describe the rate at which the isotope will decay and give off radiation.
- Using the half-life, it is possible to predict the amount of radioactive material that will remain after a given amount of time.
- Fission and fusion are two physical processes that produce massive amounts of energy from atoms.
- Fission occurs when a neutron slams into a larger atom, forcing it to excite and spilt into two smaller atoms. Additional neutrons are also released that can initiate a chain reaction.
- When each atom splits, a tremendous amount of energy is released.
- Uranium and plutonium are mostly used for fission reactions in nuclear power reactors because they are easy to initiate and control.
- The energy released by fission in these reactors’ heats water into steam. The steam is used to spin a turbine to produce carbon-free electricity.
- Fusion occurs when two atoms slam together to form a heavier atom, for example, when two hydrogen atoms fuse to form one helium atom.
- This is the same process that powers the sun and creates huge amounts of energy—several times greater than fission. In addition, it does not produce highly radioactive fission products.
- Fusion reactions are being studied by scientists but are difficult to sustain for long periods of time because of the tremendous amount of pressure and temperature needed to join the nuclei together.
Unit 1: Assessment
Suggested time to complete: 20 minutes
- Write a balanced nuclear equation to show:
- the emission of an alpha particle from thorium-[latex]\scriptsize \displaystyle 228[/latex]
- the emission of a beta particle from oxygen-[latex]\scriptsize \displaystyle 19[/latex].
- .
- State, with a reason, which type of radiation is the most ionising and which type is the least ionising.
- State, with a reason, which type of radiation is the most penetrating and which type is the least penetrating.
- Describe two ways in which exposure to excessive radiation can be harmful to living cells.
- Explain why gamma rays are more penetrating than alpha and beta particles and explain how this makes them more dangerous.
- Write a nuclear equation to show the emission of:
- an alpha particle from bismuth-[latex]\scriptsize \displaystyle 210[/latex]
- a beta particle from sodium-[latex]\scriptsize \displaystyle 24[/latex].
- Calculate the time it will take for the activity of a sample of [latex]\scriptsize _{{}}^{{131}}\text{I}[/latex], which has a half-life of [latex]\scriptsize \displaystyle 8[/latex] days, to form to [latex]\scriptsize \displaystyle 12.5\%[/latex] of its original value.
- Carbon-[latex]\scriptsize \displaystyle 14[/latex] has a half-life of [latex]\scriptsize \displaystyle 5730[/latex] years; estimate the age of a fossil which contains [latex]\scriptsize \displaystyle 12.5\%[/latex] of the carbon- [latex]\scriptsize \displaystyle 14[/latex] content of living tissue.
- Uranium-[latex]\scriptsize \displaystyle 235[/latex] can undergo fission to produce caesium-[latex]\scriptsize \displaystyle 144[/latex] and rubidium-[latex]\scriptsize \displaystyle 90[/latex]. Work out the number of neutrons released and write a nuclear equation for the reaction.
- Explain the difference between an atom bomb and a hydrogen bomb.
- Give an example of the use of radioactive tracers in:
- agriculture
- industry
- medicine
The full solutions rea at the end of the unit.
Unit: 1 Solutions
Exercise 1.1
- .
- two protons and two neutrons; atomic number decreases by [latex]\scriptsize \displaystyle 2[/latex] and mass number decreases by [latex]\scriptsize \displaystyle 4[/latex]
- an electron emitted from the nucleus; atomic number increases by [latex]\scriptsize \displaystyle 1[/latex]
- emission of alpha and beta particles reduce potential energy of nucleus; excess energy is emitted as a gamma ray
- [latex]\scriptsize \displaystyle _{{84}}^{{209}}\text{PO}\to _{\text{2}}^{\text{4}}\text{He + }_{{\text{ 82}}}^{{\text{205}}}\text{Pb}[/latex]
Exercise 1.2
- The time taken for the amount or activity of a sample to fall to half of its original value.
- [latex]\scriptsize \displaystyle 7.2\text{ mins }=\text{ }3[/latex] half-lives so it will have decayed by [latex]\scriptsize \displaystyle 87.5\%[/latex].
Exercise 1.3
- Breaking up of a nucleus into two or more smaller nuclei.
- Joining of two or more nuclei to make a single nucleus.
- Heat released when nucleus breaks up, this boils water which drives a turbine which drives a generator. Very high temperature are needed to start the fusion reaction.
Unit 1: Assessment
- .
- [latex]\scriptsize _{{90}}^{{228}}\text{Th}\to _{{88}}^{{224}}\text{Ra}+_{2}^{4}\text{He}[/latex]
- [latex]\scriptsize _{8}^{{19}}\text{O}\to _{9}^{{19}}\text{F}+_{{-1}}^{0}\text{ }\!\!\beta\!\!\text{ }[/latex]
- .
- Alpha is the most ionising – it is large and has a [latex]\scriptsize \displaystyle +2[/latex] charge. Gamma is the least ionising – it has no charge.
- Gamma is the most penetrating as it has no mass so does not collide with particles; it has no charge so is not strongly ionising. Alpha is the least penetrating because it is strongly ionising and is destroyed when it ionises another particle.
- It can kill/burn cells or cause them to mutate and become cancerous.
- They have no mass or charge so do not interact with other materials; as a result it is very difficult to protect oneself against them.
- .
- [latex]\scriptsize _{{83}}^{{210}}\text{Bi}\to _{{81}}^{{206}}\text{Tl + }_{2}^{4}\text{He}[/latex]
- [latex]\scriptsize _{{11}}^{{24}}\text{Na}\to _{{12}}^{{24}}\text{Mg + }_{{-1}}^{0}\text{ }\!\!\beta\!\!\text{ }[/latex]
- [latex]\scriptsize \displaystyle 12.5\%\text{ of value = }3\text{ half-lives }=\text{ }24\text{ days}[/latex]
- [latex]\scriptsize \displaystyle 12.5\%[/latex] is three half-lives so age = [latex]\scriptsize \displaystyle \text{5730 x 3 = 17190 years}[/latex]
- [latex]\scriptsize \displaystyle _{{92}}^{{235}}\text{U}\to _{{55}}^{{144}}\text{Cs + }_{{37}}^{{90}}\text{Rb + }_{0}^{1}\text{n}[/latex] ([latex]\scriptsize \displaystyle 1[/latex] neutron released)
- Atom bomb – energy is released because of nuclear fission. Hydrogen bomb – most energy released because of nuclear fusion.
- .
- Agriculture: monitoring how fast plants take up certain nutrients.
- Industry: identifying blockages and leaks in underground pipes.
- Medicine: locating blockages in digestive or circulatory system.
Media Attributions
- Fig 1 © DHET is licensed under a CC BY (Attribution) license
- Fig 2 © DHET is licensed under a CC BY (Attribution) license
- Fig 3 © Libre Text is licensed under a CC BY-NC-SA (Attribution NonCommercial ShareAlike) license
- Fig 4 © Libre Text is licensed under a CC BY-NC-SA (Attribution NonCommercial ShareAlike) license
- Fig 5 © all-geo.org is licensed under a CC BY-ND (Attribution NoDerivatives) license
- Fig 6 © openstax is licensed under a CC BY (Attribution) license
- Fig 7 © openstax is licensed under a CC BY (Attribution) license
- Fig 8 © openstax is licensed under a CC BY (Attribution) license
- Fig 9 © Pipodesign Philipp P Egli, is licensed under a CC BY (Attribution) license
- Fig 10 © Emoscopes is licensed under a CC BY-SA (Attribution ShareAlike) license
- Fig 11 © DHET is licensed under a CC BY (Attribution) license
- Fig 12 © DHET is licensed under a CC BY (Attribution) license
- Parts of the text in this unit were sourced from chem.libretexts.org: Introductory Chemistry, released under a CC BY NC SA licence. ↵
the process by which an unstable atomic nucleus loses energy by radiation
emitting or relating to the emission of ionising radiation or particles
the emission of ionising radiation or particles caused by the spontaneous disintegration of atomic nuclei
a process in which the nucleus of an atom is changed by being split apart or joined with the nucleus of another atom
forming an ion or causing something to form an ion
the process of forming an ion or causing something to form an ion
the time interval required for a quantity of material to decay to half its original value
a nuclear reaction in which a heavy nucleus splits spontaneously or on impact with another particle, with the release of energy
a nuclear reaction in which atomic nuclei of low atomic number fuse to form a heavier nucleus with the release of energy
